C266 – AGA 3:1992 Orifice Plate Flow Calculation

Description

This calculates the flow rate measured through an orifice plate metering system. The calculation can also be rearranged to solve the differential pressure across an orifice plate or the bore diameter.

References

AGA Report No. 3 – Orifice Metering of Natural Gas and Other Related Hydrocarbon Fluids/Manual of Petroleum Measurement Standards Chapter 14 – Natural Gas Fluids Measurement Section 3 – Concentric, Square-Edged Orifice Meters:

Part 1 – General Equations and Uncertainty Guidelines (Third Edition, September 1990)

Part 3 – Natural Gas Applications (Third Edition, August 1992)

Part 4 – Background, Development, Implementation Procedures and Subroutine Documentation (Third Edition, November 1992)

Kelton calculation reference C266

FLOCALC calculation reference F074

KIMS Calculation reference K126

Options

Fluid

Gas
Liquid

This option is used to select whether the fluid is liquid or a gas. If it is liquid the expansibility factor will be set to 1.

Solve for

Flow
Orifice size
Differential pressure

This option is used to select the desired output of the calculation.

Pressure

Measured at upstream tap (P1)
Measured at downstream tap (P2)

This option is available when solving for flow rate. It allows the user the option of inputting pressure at either the upstream or downstream tap.

Solution method

Direct substitution
Newton-Raphson

This option is used to select the method of calculation used to gain the flow rate. Both methods are generally acceptable to use though the Newton-Raphson converges faster. The direct substitution method should not be used for high viscosity liquids.

Method

Developed from the density of fluid
Developed from the real gas relative density

This option allows the user to select which set of inputs they wish to use for implementation of the AGA 3 flow rate equations. Both sets have common inputs such as geometry of the system and differential pressure, dynamic viscosity etc, but the first requires line density, the second uses real gas relative density along with PTZ data.

The remaining options will be drawn are seen in C261 or C262 and depend on the fluid used.

Calculate for

Mass
Observed volume
Standard volume
Normal volume
Energy

Calculate from

Mass
Observed volume
Standard volume
Normal volume

This option becomes active if solving for an orifice size or differential pressure

Constants

Molecular mass of air – M_air = 28.9625 g/mol

Gas constant – R = 8.314472 J/molK

Calculation

Mass flow rate

Mass flow rate as calculated ‘from the density of the fluid’ is derived from equation 3-1 in AGA 3 Part 3.

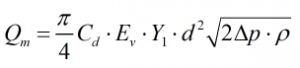
Where
C_d	=	Coefficient of discharge
E_v	=	Velocity of approach factor
Y₁	=	Expansibility factor
d	=	Orifice diameter
Δp	=	Differential pressure
ρ	=	Line density

Standard volume flow rate

Standard volume flow rate as calculated ‘from the real gas relative density’ is done derived from equation 3-6a in AGA 3 Part 3.

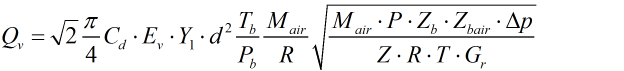
Where
C_d	=	Coefficient of discharge
E_v	=	Velocity of approach factor
Y₁	=	Expansibility factor
d	=	Orifice diameter
T_b	=	Base temperature
Pb	=	Base pressure
Mair	=	Molecular weight of air
R	=	Gas Constant
P	=	Line pressure
Z	=	Line compressibility
Zb	=	Base compressibility
Zbair	=	Base compressibility of air
T	=	Line temperature
Gr	=	Real gas relative density
Δp	=	Differential pressure

Coefficient of Discharge

AGA 3 uses the Reader-Harris Gallagher equation for the calculation of coefficient of discharge, C_d, this is derived from equations 3-11 through to 3-21 in AGA 3 Part 3 and is as follows.

Where
β	=	Diameter ratio
Re_D	=	Reynolds number
D	=	Pipe diameter in meters

Expansion Factor

AGA 3:1992 uses the Buckingham and Bean equation which is as follows:

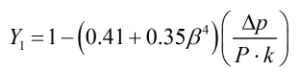
Where
P	=	Line pressure
Δp	=	Differential pressure
β	=	Diameter ratio
k	=	Isentropic exponent

Velocity of Approach Factor

The velocity of approach factor is calculated by:

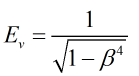
Where
β	=	Diameter ratio

Back